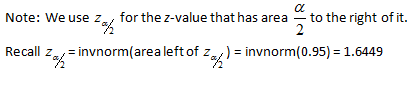
What we need to construct a 90% confidence interval:
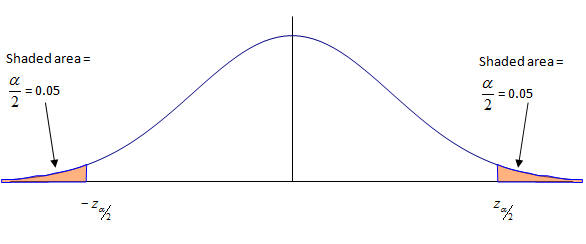
Problem: Find the z-scores in the standard normal distribution for the ends of the interval that contains the middle 90% of values.
We use the Greek letter alpha, a, for 1 - 0.9 = 0.1
If we put an area a/2 = 0.05 in each tail then the area of the region between the shaded regions will be 0.9 and hence the probability that a randomly selected
z will lie in the unshaded region is 0.99
Then we have the following picture:
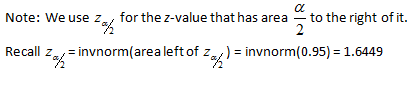
Note: It is usual to round 1.6449 to 1.645